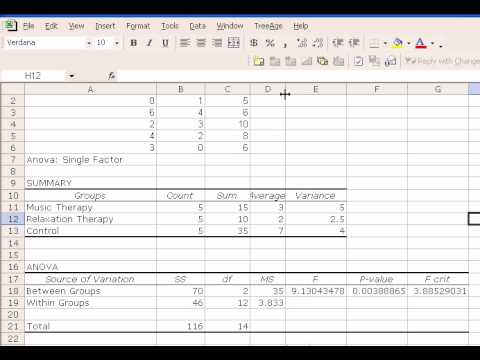
imastill.blogg.se - How to set up two way anova in excel

#HOW TO SET UP TWO WAY ANOVA IN EXCEL UPDATE#

The mean of Male is significantly greater than that of Female, from the "Dietary" table. The mean of Nor is significantly greater than that of LV and SV for factor Dietary. We can see that the Dietary and Sex are significant factors from the "Overall ANOVA" table. In the Input tab in the open dialog, clear the interactions box and click the OK button.

Click on the green lock in the ANOVA2Way1 result sheet, and select Change Parameters to open the dialog again.The data plots in the chart above suggest only a weak interaction between Sex and Dietary, so we should recalculate the effects of the two factors without interaction. We now have an Interaction Plot which can show the interaction between two factors.

#HOW TO SET UP TWO WAY ANOVA IN EXCEL UPDATE#

Click the OK button to close the dialog box and update the legend. In the Object Properties dialog box, edit the text as shown below.

Right-click on the graph legend and select Properties from the context menu.

Click the button on the 2D Graphs toolbar to create a graph.

Select the first three cells in column D and then press the Ctrl key and select the other cells in column D.

Go to the new sheet and right-click on column B and select Set As Categorical from the fly-out menu.

Right-click on the title of the "Interaction" table and select Create Copy as a new sheet from the context menu.

To further detect interaction, we can make an "Interaction Plot" using the following steps. So we can say that the main effects of both Dietary groups and Sex are significant, yet the effect on the outcome of the change in Dietary doesn't depend on the level of Sex.

Click the OK button to perform Two-Way ANOVA.įrom the "Overall ANOVA" table in the Two-Way ANOVA result sheet, we can see that Dietary and Sex are both significant factors, but the interaction between them is not significant.

In the Means Comparison tab, set Significance Level to 0.05, and check the box beside Tukey to select this test as the means comparison method.

In the Descriptive statistics tab, check all boxes.

Also in the Input tab, select column A,B and C for Factor A, Factor B and Data, respectively.Open the Two-Way ANOVA dialog by choosing the menu item Statistics: ANOVA: Two-Way ANOVA, then in the Input tab, set the Input Data mode as Indexed.Start with a new workbook and import the file \ Samples\ Statistics\ SBP_Index.dat.One approach to solve this problem is to construct a two-way ANOVA model predicting mean SBP level. Sex and dietary group may be independent or they may interact with each other. Researchers are interested in the effects of sex and dietary group on systolic blood pressure (SBP) The "dietary group" factor includes three groups: strict vegetarians (SV) who eat no animal products of any kind lacto vegetarians (LV) who eat dairy products but no other animal products and "normal" (NOR) subjects who eat a standard American diet. Minimum Origin Version Required: Origin 2016 SR0 What you will learn Two way ANOVA is an appropriate method to analyze the main effects of and interactions between two factors. The effects on the outcome of the change in one factor may depend on the level of the other factor, so we need to consider the interactions between two factors. In some cases, we want to examine the relationship between two factors (categorical variables) and a continuous outcome variable.